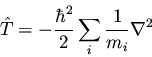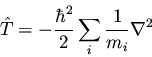Next: The Born-Oppenheimer Approximation
Up: Molecular Quantum Mechanics
Previous: Molecular Quantum Mechanics
We have noted before that the kinetic energy for a system of particles
is
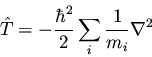 |
(132) |
The potential energy for a system of charged particles is
 |
(133) |
For a molecule, it is reasonable to split the kinetic energy into two
summations--one over electrons, and one over nuclei. Similarly, we
can split the potential energy into terms representing interactions
between nuclei, between electrons, or between electrons and nuclei.
Using i and j to index electrons, and A and B to index nuclei,
we have (in atomic units)
 |
(134) |
where
 ,
,
 ,
and
,
and
 .
This is known as the
``exact'' nonrelativistic Hamiltonian in field-free space. However,
it is important to remember that this Hamiltonian neglects at least
two effects. Firstly, although the speed of an electron in a hydrogen
atom is less than 1% of the speed of light, relativistic mass
corrections can become appreciable for the inner electrons of heavier
atoms. Secondly, we have neglected the spin-orbit effects. From the
point of view of an electron, it is being orbited by a nucleus which
produces a magnetic field (proportional to L); this field interacts
with the electron's magnetic moment (proportional to S), giving rise
to a spin-orbit interaction (proportional to
.
This is known as the
``exact'' nonrelativistic Hamiltonian in field-free space. However,
it is important to remember that this Hamiltonian neglects at least
two effects. Firstly, although the speed of an electron in a hydrogen
atom is less than 1% of the speed of light, relativistic mass
corrections can become appreciable for the inner electrons of heavier
atoms. Secondly, we have neglected the spin-orbit effects. From the
point of view of an electron, it is being orbited by a nucleus which
produces a magnetic field (proportional to L); this field interacts
with the electron's magnetic moment (proportional to S), giving rise
to a spin-orbit interaction (proportional to
 for a diatomic.) Although spin-orbit effects can be important, they
are generally neglected in quantum chemical calculations.
for a diatomic.) Although spin-orbit effects can be important, they
are generally neglected in quantum chemical calculations.




Next: The Born-Oppenheimer Approximation
Up: Molecular Quantum Mechanics
Previous: Molecular Quantum Mechanics