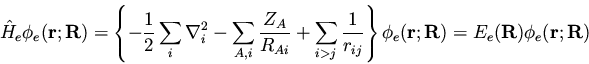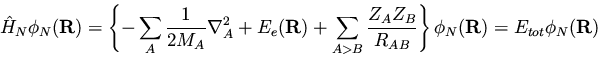The Born-Oppenheimer Approximation

Next: Separation of the Nuclear Up: Molecular Quantum Mechanics Previous: The Molecular Hamiltonian

The Born-Oppenheimer Approximation

We know that if a Hamiltonian is separable into two or more terms, then the total eigenfunctions are products of the individual eigenfunctions of the separated Hamiltonian terms, and the total eigenvalues are sums of individual eigenvalues of the separated Hamiltonian terms.

Consider, for example, a Hamiltonian which is separable into two terms, one involving coordinate q₁ and the other involving coordinate q₂.

$\begin{displaymath}\hat{H} = \hat{H}_1(q_1) + \hat{H}_2(q_2) \end{displaymath}$

(135)

with the overall Schrödinger equation being

$\begin{displaymath}\hat{H} \psi(q_1, q_2) = E \psi(q_1, q_2) \end{displaymath}$

(136)

If we assume that the total wavefunction can be written in the form $\psi(q_1, q_2) = \psi_1(q_1) \psi_2(q_2)$ , where $\psi_1(q_1)$ and $\psi_2(q_2)$ are eigenfunctions of $\hat{H}_1$ and $\hat{H}_2$ with eigenvalues E₁ and E₂, then

$\displaystyle \hat{H} \psi(q_1, q_2)$	=	$\displaystyle ( \hat{H}_1 + \hat{H}_2 ) \psi_1(q_1) \psi_2(q_2)$	(137)
	=	$\displaystyle \hat{H}_1 \psi_1(q_1) \psi_2(q_2) + \hat{H}_2 \psi_1(q_1) \psi_2(q_2)$
	=	$\displaystyle E_1 \psi_1(q_1) \psi_2(q_2) + E_2 \psi_1(q_1) \psi_2(q_2)$
	=	$\displaystyle (E_1 + E_2) \psi_1(q_1) \psi_2(q_2)$
	=	$\displaystyle E \psi(q_1, q_2)$

Thus the eigenfunctions of $\hat{H}$ are products of the eigenfunctions of $\hat{H}_1$ and $\hat{H}_2$ , and the eigenvalues are the sums of eigenvalues of $\hat{H}_1$ and $\hat{H}_2$ .

If we examine the nonrelativistic Hamiltonian (134), we see that the term

$\begin{displaymath}\sum_{Ai} \frac{Z_A}{r_{Ai}} \end{displaymath}$

(138)

prevents us from cleanly separating the electronic and nuclear coordinates and writing the total wavefunction as $\psi({\bf r}, {\bf R}) = \psi_e({\bf r}) \psi_N({\bf R})$ , where ${\bf r}$ represents the set of all electronic coordinates, and ${\bf R}$ represents the set of all nuclear coordinates. The Born-Oppenheimer approximation is to assume that this separation is nevertheless approximately correct.

Qualitatively, the Born-Oppenheimer approximation rests on the fact that the nuclei are much more massive than the electrons. This allows us to say that the nuclei are nearly fixed with respect to electron motion. We can fix ${\bf R}$ , the nuclear configuration, at some value ${\bf R_a}$ , and solve for $\psi_e ({\bf r}; {\bf R_a})$ ; the electronic wavefunction depends only parametrically on ${\bf R}$ . If we do this for a range of ${\bf R}$ , we obtain the potential energy curve along which the nuclei move.

We now show the mathematical details. Let us abbreviate the molecular Hamiltonian as

$\begin{displaymath} \hat{H} = \hat{T}_N({\bf R}) + \hat{T}_e({\bf r}) + \hat{V... ... + \hat{V}_{eN}({\bf r}, {\bf R}) + \hat{V}_{ee}({\bf r}) \end{displaymath}$

(139)

where the meaning of the individual terms should be obvious. Initially, $\hat{T}_N({\bf R})$ can be neglected since $\hat{T}_N$ is smaller than $\hat{T}_e$ by a factor of M_A / m_e, where m_e is the mass of an electron. Thus for a fixed nuclear configuration, we have

$\begin{displaymath}\hat{H}_{el} = \hat{T}_e({\bf r}) + \hat{V}_{eN}({\bf r}; {\bf R}) + \hat{V}_{NN}({\bf R}) + \hat{V}_{ee}({\bf r}) \end{displaymath}$

(140)

such that

$\begin{displaymath} \hat{H}_{el} \phi_e({\bf r}; {\bf R}) = E_{el} \phi_e({\bf r}; {\bf R}) \end{displaymath}$

(141)

This is the ``clamped-nuclei'' Schrödinger equation. Quite frequently $\hat{V}_{NN}({\bf R})$ is neglected in the above equation, which is justified since in this case ${\bf R}$ is just a parameter so that $\hat{V}_{NN}({\bf R})$ is just a constant and shifts the eigenvalues only by some constant amount. Leaving $\hat{V}_{NN}({\bf R})$ out of the electronic Schrödinger equation leads to a similar equation,

$\begin{displaymath}\hat{H}_e = \hat{T}_e({\bf r}) + \hat{V}_{eN}({\bf r};{\bf R}) + \hat{V}_{ee}({\bf r}) \end{displaymath}$

(142)

$\begin{displaymath} \hat{H}_e \phi_e({\bf r};{\bf R}) = E_e \phi_e({\bf r};{\bf R}) \end{displaymath}$

(143)

where we have used a new subscript ``e'' on the electronic Hamiltonian and energy to distinguish from the case where $\hat{V}_{NN}$ is included.

We now consider again the original Hamiltonian (139). If we insert a wavefunction of the form $\phi_T({\bf r}, {\bf R}) = \phi_e({\bf r};{\bf R}) \phi_N({\bf R})$ , we obtain

$\begin{displaymath}\hat{H} \phi_e({\bf r};{\bf R})\phi_N({\bf R})= E_{tot} \phi_e({\bf r};{\bf R})\phi_N({\bf R}) \end{displaymath}$

(144)

$\begin{displaymath} \{\hat{T}_N({\bf R}) + \hat{T}_e({\bf r}) + \hat{V}_{eN}({\b... ...hi_N({\bf R})= E_{tot} \phi_e({\bf r};{\bf R})\phi_N({\bf R}) \end{displaymath}$

(145)

Since $\hat{T}_e$ contains no ${\bf R}$ dependence,

$\begin{displaymath}\hat{T}_e \phi_e({\bf r};{\bf R})\phi_N({\bf R})= \phi_N({\bf R})\hat{T}_e \phi_e({\bf r};{\bf R}) \end{displaymath}$

(146)

However, we may not immediately assume

$\begin{displaymath}\hat{T}_N \phi_e({\bf r};{\bf R})\phi_N({\bf R})= \phi_e({\bf r};{\bf R})\hat{T}_N \phi_N({\bf R}) \end{displaymath}$

(147)

(this point is tacitly assumed by most introductory textbooks). By the chain rule,

$\begin{displaymath}\nabla^2_A \phi_e({\bf r};{\bf R})\phi_N({\bf R})= \phi_e({\b... ..._N({\bf R})+ \phi_N({\bf R})\nabla^2_A \phi_e({\bf r};{\bf R}) \end{displaymath}$

(148)

Using these facts, along with the electronic Schrödinger equation,

$\begin{displaymath}\{\hat{T}_e + \hat{V}_{eN}({\bf r};{\bf R}) + \hat{V}_{ee}(... ...\hat{H}_e \phi_e({\bf r};{\bf R})= E_e \phi_e({\bf r};{\bf R}) \end{displaymath}$

(149)

we simplify (145) to

$\displaystyle \phi_e({\bf r};{\bf R})\hat{T}_N \phi_N({\bf R})+ \phi_N({\bf R})\phi_e({\bf r};{\bf R})(E_e + \hat{V}_{NN})$			(150)
$\displaystyle - \left\{ \sum_A \frac{1}{2M_A} (2\nabla_A \phi_e({\bf r};{\bf R}... ..._A \phi_N({\bf R})+ \phi_N({\bf R})\nabla^2_A \phi_e({\bf r};{\bf R})) \right\}$
	=	$\displaystyle E_{tot} \phi_e({\bf r};{\bf R})\phi_N({\bf R})$

We must now estimate the magnitude of the last term in brackets. Following Steinfeld [5], a typical contribution has the form $1/(2M_A) \nabla^2_A \phi_e({\bf r};{\bf R})$ , but $\nabla_A \phi_e({\bf r};{\bf R})$ is of the same order as $\nabla_i \phi_e({\bf r};{\bf R})$ since the derivatives operate over approximately the same dimensions. The latter is $\phi_e({\bf r}; {\bf R}) p_e$ , with p_e the momentum of an electron. Therefore $1/(2M_A) \nabla^2_A \phi_e({\bf r};{\bf R})\approx p_e^2/(2M_A) = (m/M_A)E_e$ . Since $m/M_A \sim 1/10000$ , the term in brackets can be dropped, giving

$\begin{displaymath}\phi_e({\bf r};{\bf R})\hat{T}_N \phi_N({\bf R})+ \phi_N({\bf... ... r};{\bf R})= E_{tot} \phi_e({\bf r};{\bf R})\phi_N({\bf R}) \end{displaymath}$

(151)

$\begin{displaymath}\{\hat{T}_N + E_e + \hat{V}_{NN} \} \phi_N({\bf R})= E_{tot} \phi_N({\bf R}) \end{displaymath}$

(152)

This is the nuclear Shrodinger equation we anticipated--the nuclei move in a potential set up by the electrons.

To summarize, the large difference in the relative masses of the electrons and nuclei allows us to approximately separate the wavefunction as a product of nuclear and electronic terms. The electronic wavefucntion $\phi_e({\bf r};{\bf R})$ is solved for a given set of nuclear coordinates,

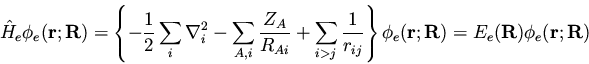$\begin{displaymath}\hat{H}_e \phi_e({\bf r};{\bf R})= \left\{ -\frac{1}{2} \sum_... ... \phi_e({\bf r};{\bf R})= E_e({\bf R}) \phi_e({\bf r};{\bf R}) \end{displaymath}$

(153)

and the electronic energy obtained contributes a potential term to the motion of the nuclei described by the nuclear wavefunction $\phi_N({\bf R})$ .

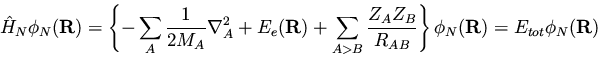$\begin{displaymath}\hat{H}_N \phi_N({\bf R})= \left\{ -\sum_A \frac{1}{2M_A} \na... ...Z_B}{R_{AB}} \right\} \phi_N({\bf R})= E_{tot} \phi_N({\bf R}) \end{displaymath}$

(154)

As a final note, many textbooks, including Szabo and Ostlund [4], mean total energy at fixed geometry when they use the term ``total energy'' (i.e., they neglect the nuclear kinetic energy). This is just E_el of equation (141), which is also E_e plus the nuclear-nuclear repulsion. A somewhat more detailed treatment of the Born-Oppenheimer approximation is given elsewhere [6].

Next: Separation of the Nuclear Up: Molecular Quantum Mechanics Previous: The Molecular Hamiltonian